Hyperfocal Distance a Short Introduction by Christopher John Ball
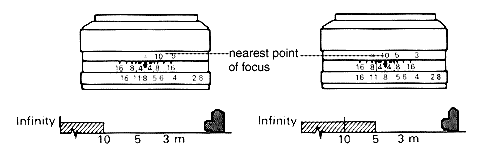
When a lens is focused on infinity, the depth of field extends from infinity towards a point nearer the camera lens. This distance from the camera to the near limit of sharp field is called the Hyperfocal distance.
If you then focus on the Hyperfocal distance, the depth of field extends from half the Hyperfocal distance to infinity.
As the above diagram shows, to exploit the ' extra ' depth of field available for a given aperture, set your lens to infinity. Then note, via the depth of field scale on the lens, the nearest distance still within the depth of field for the chosen aperture. In the above example this is 10m when using f8. This is the ' Hyperfocal ' distance for this combination. Now refocus the lens by moving the focusing ring markings so that the focus point is at 10m ( the Hyperfocal distance ). Depth of field will now extend from half this distance towards infinity. This method of focusing is often exploited in the manufacture of cheap point and shoot camera's, but it does have uses within more advanced photography.
July 2024 Several books, featuring Christopher John Ball's photographs, are now available through Amazon or click on an image below to purchase via secure payments on lulu.com

Tweet